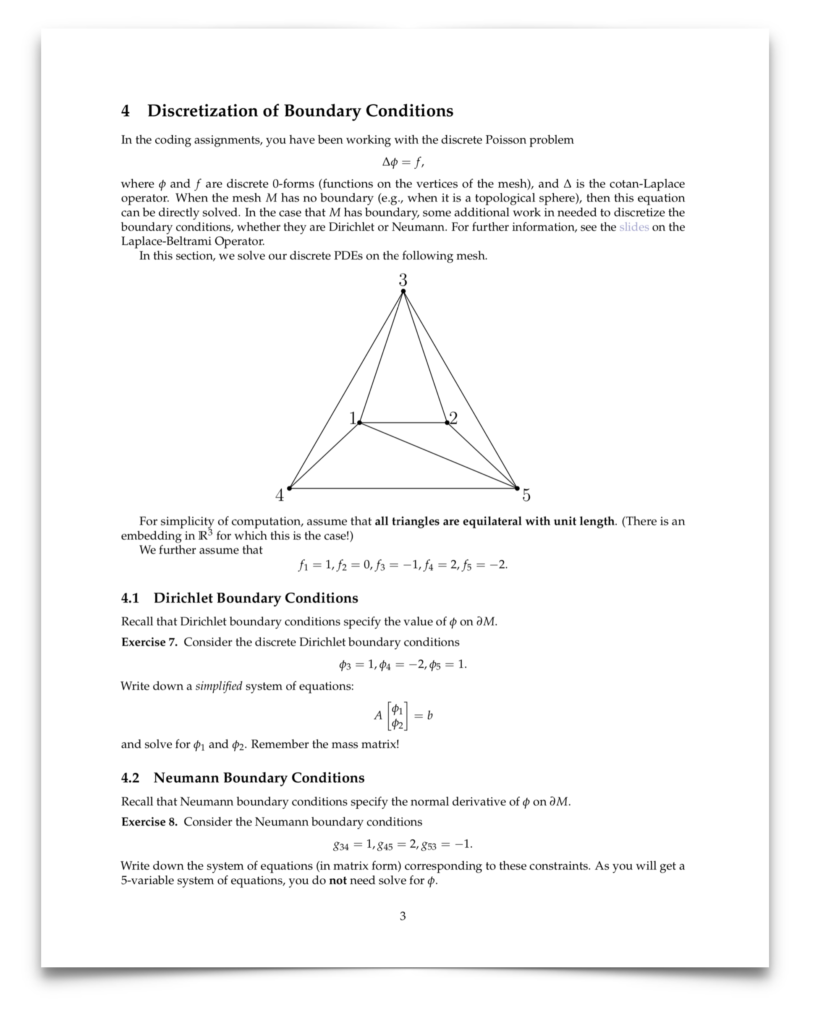
Here’s the writeup for your last assignment, which plays the role of the final in the course. (Note that there is no final exam!)
Note: For the final assignment, you can do either this assignment or A6. Overall, you just need to be sure you completed A0 and A1, as well as 3 of the assignments A2–A6 (your choice which ones. See this Piazza post for more details.) Also, you cannot use late days on this assignment since it’s the last one.
This time, we’re taking off the “training wheels” and having you read a real paper, rather than course notes. Why? Because you’re ready for it! At this point you have all the fundamental knowledge you need to go out into the broader literature and start implementing all sorts of algorithms that are built on top of ideas from differential geometry. In fact, this particular algorithm is not much of a departure from things you’ve done already: solving simple equations involving the Laplacian on triangle meshes. As discussed in our lecture on the Laplacian, you’ll find many algorithms in digital geometry processing that have this flavor: compute some basic data (e.g., using a local formula at each vertex), solve a Laplace-like equation, compute some more basic data, and so on.
Your main references for this assignment will be:
- this video, which gives a brief (18-minute) overview of the algorithm, and
- this paper, which explains the algorithm in detail.
Written exercises for this assignment are found below. Submit your responses to Gradescope by 5:59pm ET, May 21, 2021.