The written portion of Assignment 2 can be found
here. It takes a look at the curvature of smooth and discrete surfaces, filling in some of the details from lecture. Note that there are a fair number of exercises here, so it might be wise to split them up over the course of the assignment.
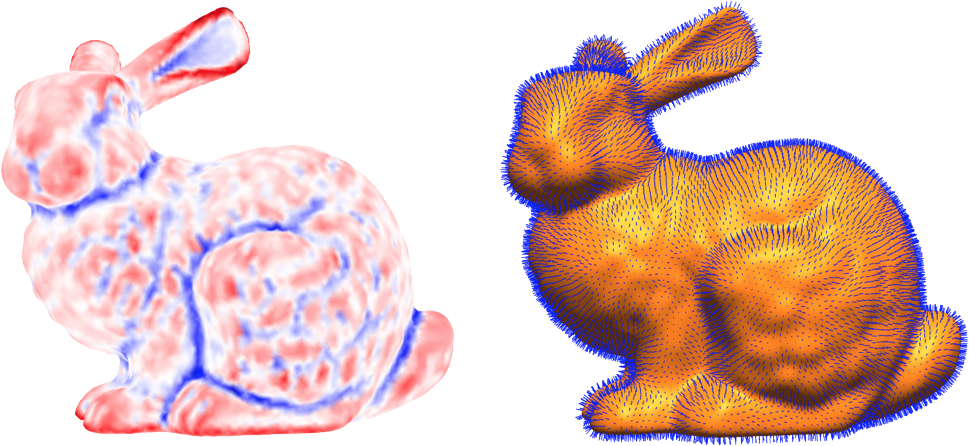
For the coding portion of this assignment, you will implement various expressions for discrete curvatures and surfaces normals that you will derive in the written assignment. (However, the final expressions are given below in case you want to do the coding first.) Once implemented, you will be able to visualize these geometric quantities on a mesh. For simplicity, you may assume that the mesh has no boundary.
Getting Started
Please implement the following routines in
core/geometry.[js/cpp]:
- angle
- dihedralAngle
- vertexNormalAngleWeighted
- vertexNormalSphereInscribed
- vertexNormalAreaWeighted
- vertexNormalGaussianCurvature
- vertexNormalMeanCurvature
- angleDefect
- totalAngleDefect
- scalarMeanCurvature
- circumcentricDualArea
- principalCurvatures
Notes
1. The dihedral angle between the normals \(N_{ijk}\) and \(N_{ijl}\) of two adjacent faces \(ijk\) and \(ijl\) (respectively) is given by
\[ \theta_{ij} := \text{atan2}\left(\frac{e_{ij}}{\|e_{ij}\|} \cdot \left(N_{ijk} \times N_{jil}\right), N_{ijk} \cdot N_{jil}\right) \]
where \(e_{ij}\) is the vector from vertex \(i\) to vertex \(j\).
2. The formulas for the angle weighted normal, sphere inscribed normal, area weighted normal, discrete Gaussian curvature normal and discrete mean curvature normal at vertex \(i\) are
\[\begin{aligned}
N_i^\phi &:= \sum_{ijk \in F} \phi_i^{jk}N_{ijk}\\
N_i^S &:= \sum_{ijk \in F} \frac{e_{ij} \times e_{ik}} {\|e_{ij}\|^2\|e_{ik}\|^2}\\
N_i^A &:= \sum_{ijk \in F} A_{ijk}N_{ijk}\\
KN_i &= \frac 12 \sum_{ij \in E} \frac{\theta_{ij}}{\|e_{ij}\|}e_{ij}\\
HN_i &= \frac 12 \sum_{ij \in E}\left(\cot\left(\alpha_k^{ij}\right) + \cot\left(\beta_l^{ij}\right)\right)e_{ij}
\end{aligned}
\]
where \(\phi_i^{jk}\) is the interior angle between edges \(e_{ij}\) and \(e_{ik}\), and \(A_{ijk}\) is the area of face \(ijk\). Note that sums are taken only over elements (edges or faces) containing vertex \(i\). Normalize the final value of all your normal vectors before returning them.
3. The circumcentric dual area at vertex \(i\) is given by
\[A_i := \frac 18 \sum_{ijk \in F} \|e_{ik}\|^2\cot\left(\alpha_j^{ki}\right) + \|e_{ij}\|^2\cot\left(\beta_k^{ij}\right)\]
4. The discrete scalar Gaussian curvature (also known as angle defect) and discrete scalar mean curvature at vertex \(i\) are given by
\[\begin{aligned}
K_i &:= 2\pi - \sum_{ijk \in F} \phi_i^{jk}\\
H_i &:= \frac 12 \sum_{ij \in E} \theta_{ij}\|e_{ij}\|
\end{aligned}
\]
Note that these quantities are discrete 2-forms, i.e., they represent the total Gaussian and mean curvature integrated over a neighborhood of a vertex. They can be converted to pointwise quantities (i.e., discrete 0-forms at vertices) by dividing them by the circumcentric dual area of the vertex (i.e., by applying the discrete Hodge star).
5. You are required to derive expressions for the principal curvatures \(\kappa_1\) and \(\kappa_2\) in exercise 4 of the written assignment. Your implementation of
principalCurvatures should return the (pointwise) minimum and maximum principal curvature values at a vertex (in that order).
Submission Instructions
Please submit only the source code file
geometry.js or
geometry.cpp (depending on whether you’re using JavaScript or C++). This means your code should not depend on edits made to any other files. Then follow the usual hand-in instructions.
Handin instructions can be found in the Assignments section of the main page.
