Your next reading covers one of the most fundamental objects in differential geometry, and one of the most useful objects in practical geometry processing: the Laplace-Beltrami operator \(\Delta\), which we'll often refer to as just the “Laplacian”. This operator generalizes the familiar Laplace operator \(\Delta = \frac{\partial^2}{\partial x_1^2} + \cdots + \frac{\partial^2}{\partial x_n^2}\) from Euclidean \(\mathbb{R}^n\) to general curved manifolds. Like the ordinary Laplacian, at a very basic level Laplace-Beltrami provides information about the "curvature" of a function. It also shows up in an enormous number of physical and geometric equations, and for this reason there has been intense study of different ways to discretize the Laplacian (not only for simplicial meshes, but also point clouds and other discrete surface representations). The reading will expose you to some of the key issues to think about when designing a discrete Laplacian. For this reading, you can choose either of the following two papers:

• Wardetzky, Mathur, Kälberer, Grinspun, “Discrete Laplace Operators: No Free Lunch” (2007)
• Bobenko & Springborn, “A Discrete Laplace-Beltrami Operator for Simplicial Surfaces” (2007)
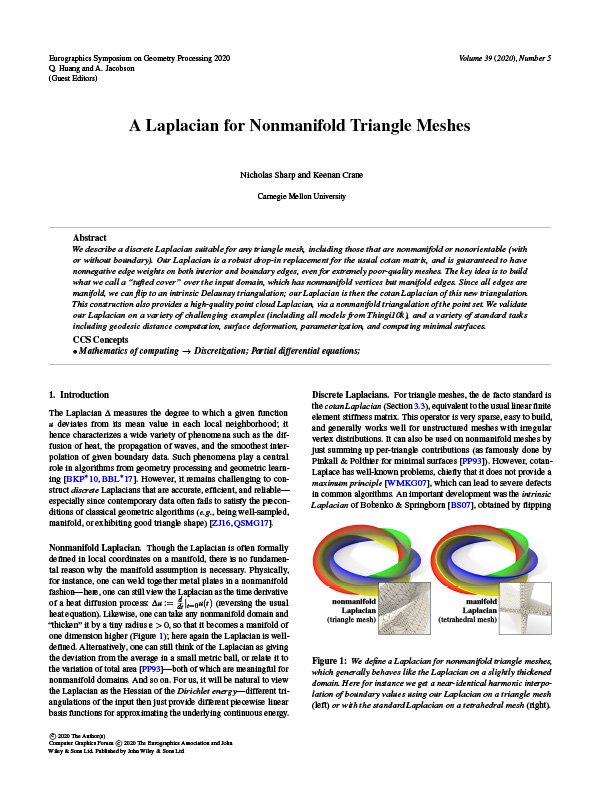
• Sharp & Crane, “A Discrete Laplace-Beltrami Operator for Simplicial Surfaces” (2020)

You should not worry about deeply understanding all of the mathematical details in these papers; the point is just to get a sense of the issues at stake, and how these considerations translate into practical definitions of discrete Laplace matrices. The first paper, by Wardetzky et al, considers a “No Free Lunch” theorem for discrete Laplacians that continues our story of “The Game” played in discrete differential geometry. The second paper, by Bobenko & Springborn, considers the important perspective of intrinsic triangulations of polyhedral surfaces, and uses this perspective to develop a Laplace operator that is well-behaved even for very poor quality triangulations. The third paper, by Sharp & Crane, defines a well-behaved Laplacian for general triangle meshes, rather than just those that satisfy the manifold assumption. As usual, you should write a short 2-3 sentence summary, plus one question about something you didn't understand or want to know more about. Handin instructions can be found in the Readings section of the main page.